How to Calculate Chi Square in Excel?
Are you looking for a quick and easy way to calculate chi square in Excel? If so, you’ve come to the right place. In this article, we’ll walk you through the steps of calculating chi square in Microsoft Excel. We’ll include a simple example to illustrate the process and provide guidance on interpreting the results. By the end of this article, you’ll have the confidence and knowledge to calculate chi square in Excel. Let’s get started!
Chi Square in Excel can be calculated using the CHITEST function. This function is used to compare two sets of observed data. To calculate Chi Square in Excel:
- Enter the observed data into two columns in the spreadsheet.
- In an empty cell, type the CHITEST function, which is: =CHITEST(OBSERVED_RANGE1, OBSERVED_RANGE2).
- Replace OBSERVED_RANGE1 and OBSERVED_RANGE2 with the cell ranges for the two sets of data.
- Hit Enter. The CHITEST function will return the Chi Square value.
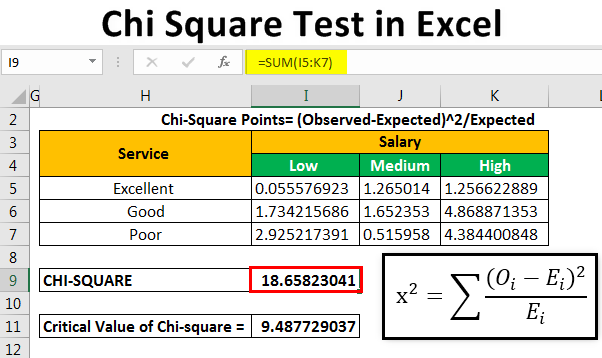
Introduction to Chi Square Calculation
Chi Square is a statistical test used to compare observed data with expected data. It is used to determine whether there is a significant difference between the two sets of data. Chi Square can also be used to test hypotheses about the distribution of categorical variables. This article will provide an overview of how to calculate Chi Square in Microsoft Excel.
Understanding Chi Square
Chi Square is a statistical test used to compare observed data with expected data. The test is based on the chi-squared statistic, which measures the difference between observed and expected frequencies in a given sample. The statistic is calculated by subtracting the expected frequency from the observed frequency, then dividing the result by the expected frequency and squaring the result. The chi-square statistic is then compared to the critical value of the chi-square distribution. If the calculated value is greater than the critical value, the null hypothesis is rejected, and the difference between the observed and expected frequencies is deemed to be statistically significant.
Chi Square is used to test hypotheses about the distribution of categorical variables. It is used to determine whether there is a significant difference between two sets of data, such as the results of a survey or the outcome of an experiment. The test is used to compare the observed frequencies of two variables (for example, a group of survey respondents who said they preferred one type of product over another) with the expected frequencies (the proportion of the population that would be expected to prefer that type of product).
Calculating Chi Square in Excel
Calculating Chi Square in Microsoft Excel is relatively easy. To do this, you will need to enter the observed and expected frequencies into an Excel spreadsheet and then use the CHISQ.DIST function to calculate the chi-squared statistic.
The CHISQ.DIST function takes three arguments: the observed frequency, the expected frequency, and the degree of freedom. The degree of freedom is determined by the number of categories in the data set. For example, if there are two categories (e.g. male and female), the degree of freedom is one.
Steps to Calculate Chi Square in Excel
The first step in calculating Chi Square in Excel is to enter the observed and expected frequencies into an Excel spreadsheet. The observed frequencies should be entered in one column, while the expected frequencies should be entered in another.
The next step is to use the CHISQ.DIST function to calculate the chi-squared statistic. This function takes three arguments: the observed frequency, the expected frequency, and the degree of freedom. To use the function, enter the observed and expected frequencies into the function, and then enter the degree of freedom.
Interpreting the Results
Once the CHISQ.DIST function has been used to calculate the chi-squared statistic, the result can be interpreted. If the calculated value is greater than the critical value of the chi-square distribution, then the null hypothesis is rejected, and the difference between the observed and expected frequencies is deemed to be statistically significant.
Using the Results
The results of the chi-squared test can be used in a variety of ways. For example, if the test is used to compare the results of a survey, it can be used to determine whether there is a significant difference between the preferences of different groups of respondents. It can also be used to test hypotheses about the distribution of categorical variables, such as whether a particular variable is evenly distributed across a population.
Frequently Asked Questions
What is a Chi Square Test?
A Chi Square test is a statistical test used to determine if there is a significant difference between the expected frequencies and the observed frequencies in one or more categories. It is also used to test relationships between two categorical variables. The Chi Square test is used to compare observed data with expected data to see if there is a significant difference between them.
What is the Formula for a Chi Square Test?
The formula for a Chi Square test is: X2 = Σ , where O is the observed frequency and E is the expected frequency. The summation is done over all categories. The result of the formula is the Chi Square statistic, which can then be used to determine the significance of the difference between the observed and expected frequencies.
How to Calculate Chi Square in Excel?
To calculate the Chi Square statistic in Excel, you will need to enter the observed and expected frequencies into the appropriate cells. First, enter the observed frequencies into the first column. Then, enter the expected frequencies into the second column. Next, select the two columns of data and choose the “Data Analysis” option from the “Data” tab. Finally, select the “Chi-Square Test” option and click “OK”. The resulting Chi Square statistic will be displayed in the “Output” tab.
What is the Significance of the Chi Square Statistic?
The significance of the Chi Square statistic is determined by comparing it to a critical value from the Chi Square distribution table. If the calculated statistic is greater than the critical value, then it is considered to be significant and the difference between the observed and expected frequencies is not due to chance. If the calculated statistic is less than the critical value, then it is considered to be not significant and the difference between the observed and expected frequencies is considered to be due to chance.
What are the Assumptions of a Chi Square Test?
The assumptions of a Chi Square test include that the data must be categorical, that the expected frequencies should be greater than 5 in each category, that the expected frequencies should be at least 10% of the total observations, and that the observations are independent.
What are the Limitations of a Chi Square Test?
The limitations of a Chi Square test include that it is not suitable for highly skewed distributions, that it is sensitive to sample size, and that it cannot be used to determine the direction of the relationship between two categorical variables. Additionally, the Chi Square test is not appropriate for testing relationships between variables that are not categorical.
How to Calculate Chi Square Using Excel =CHISQ.TEST and =CHISQ.INV.RT
In conclusion, calculating Chi Square in Excel is a relatively straightforward process. With just a few clicks, you can calculate the Chi Square statistic in Excel and use it to analyze relationships between two or more variables. Whether you’re a student or a professional researcher, understanding how to calculate Chi Square in Excel is an important skill to have in your data analysis toolbox.




















